为了加强学术交流与合作研究, 了解偏微分方程的最新研究成果和发展动态,由77779193永利官网王春朋教授提议,77779193永利官网和东北大学数学系联合组织,将于2022年1月5日举办”2022年非线性偏微分方程”线上学术会议。本次会议邀请偏微分方程领域的优秀学者, 围绕非线性偏微分方程前沿进展展开交流和讨论, 为青年教师和研究生提供学习和交流的平台。
会议组织者:
王春朋(主席) (77779193永利官网), 刘长春(77779193永利官网)
郭斌(77779193永利官网), 赵晓朋(东北大学), 段宁(东北大学)
线上腾讯会议:
会议ID: 921-809-822
会议链接: https://meeting.tencent.com/dm/MkjneVedoAKU
校内联系人:郭斌 bguo@jlu.edu.cn
日程表:
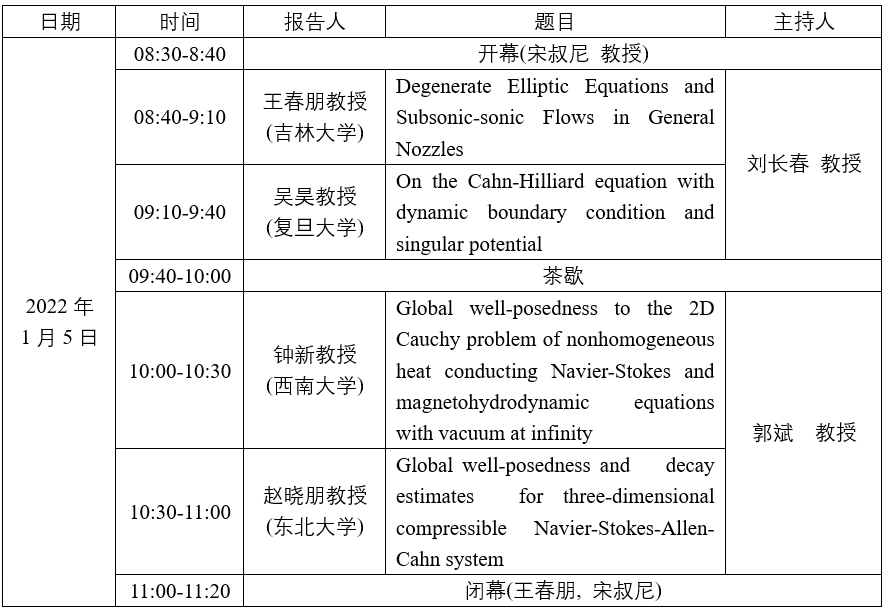
报告题目与摘要:
Degenerate Elliptic Equations and Subsonic-sonic Flows in General Nozzles
王春朋(77779193永利官网)
This talk concerns subsonic-sonic potential flows in two dimensional nozzles, which are governed by nonlinear degenerate elliptic equations. For finitely long symmetric nozzles, it is shown that there exists a unique Lipschitz continuous subsonic-sonic flow, and the location of sonic points is classified completely.
报告人:王春朋, 77779193永利官网教授、国家杰出青年基金获得者、全国优秀百篇博士学位论文获得者、国务院特殊津贴专家,从事退化抛物方程和椭圆-双曲混合型偏微分方程方面的研究。近年来主要围绕光滑跨音速流理论研究拟线性混合型偏微分方程的适定性,在ARMA、Adv. Math., CPDE, JDE, SIAM J. Math. Anal., SIAM J. Control Optim. 等著名期刊上发表了系列重要论文。
On the Cahn-Hilliard equation with dynamic boundary condition and singular potential
吴昊(复旦大学)
We report some recent progresses on the Cahn-Hilliard equation with dynamic boundary conditions, which accounts for nontrivial short-range interactions of the binary mixture near the boundary. When the potentials take the form of physically relevant singular type, we show the well-posedness and long-time behavior of the initial boundary value problem.
报告人:吴昊, 复旦大学数学科学学院教授,博士生导师,教育部青年长江学者,上海市青年拔尖人才,主要从事偏微分方程理论方面的研究。吴教授曾主持国家自然科学基金项目,上海市自然科学基金项目等多项,荣获第二届中国工业与应用数学会优秀青年学者奖等,在ARMA,MMMAS,Ann. Inst. H. Poincare Anal. Non Lineaire,SIAM J. Math. Anal., Calc. Var. PDE,European J. Appl. Math., JDE等杂志发表了系列重要论文。
Global well-posedness to the 2D Cauchy problem of nonhomogeneous heat conducting Navier-Stokes and magnetohydrodynamic equations with vacuum at infinity
钟新(西南大学)
In this talk, we investigate the 2D Cauchy problem of nonhomogeneous heat conducting magnetohydrodynamic equations in the whole plane. For the initial density allowing vacuum at infinity, we derive the global existence and uniqueness of strong solutions provided that the initial density and the initial magnetic decay not too slowly at infinity. In particular, the initial data can be arbitrarily large. The method is based on delicate spatial weighted estimates and the structural characteristic of the system under consideration. As a byproduct, we get the global existence of strong solutions to the 2D Cauchy problem for nonhomogeneous heat conducting Navier-Stokes equations with vacuum at infinity.
报告人:钟新,西南大学数学与统计学院教授,重庆英才青年拔尖人才,重庆市巴渝学者青年学者。主要研究兴趣为流体方程组解的整体适定性和奇点的形成,部分研究成果发表在 J. Math. Pures Appl.、Indiana Univ. Math. J.、Calc. Var. Partial Differential Equations、J. Math. Fluid Mech.、Nonlinearity、J. Differential Equations 等数学期刊上。主持完成或在研国家自然科学基金、中国博士后科学基金、重庆市自然科学基金等国家级和省部级科研项目8项。
Global well-posedness and decay estimates for three-dimensional compressible Navier-Stokes-Allen-Cahn system
赵晓朋(东北大学)
We study the small data global well-posedness and time-decay rates of solutions to the Cauchy problem for 3D compressible Navier-Stokes-Allen-Cahn equations via a refined pure energy method. In particular, the optimal decay rates of the higher order spatial derivatives of the solution are obtained, the

negative Sobolev norms is shown to be preserved along time evolution and enhance the decay rates.
报告人: 赵晓朋, 东北大学数学系副教授, 硕士生导师, 沈阳市拔尖人才, 主要从事非线性发展方程理论方面的研究, 部分研究成果发表在J. Nonlinear Sci.、Proc. Roy. Soc. Edinburgh Sect. A、J. Hyperbolic Diff. Equ.、Topol. Method. Nonlinear Sci.、Adv. Differential Equations等数学期刊上。 主持完成国家自然科学基金、江苏省自然科学基金和中国博士后基金等国家级和省部级科研项目。